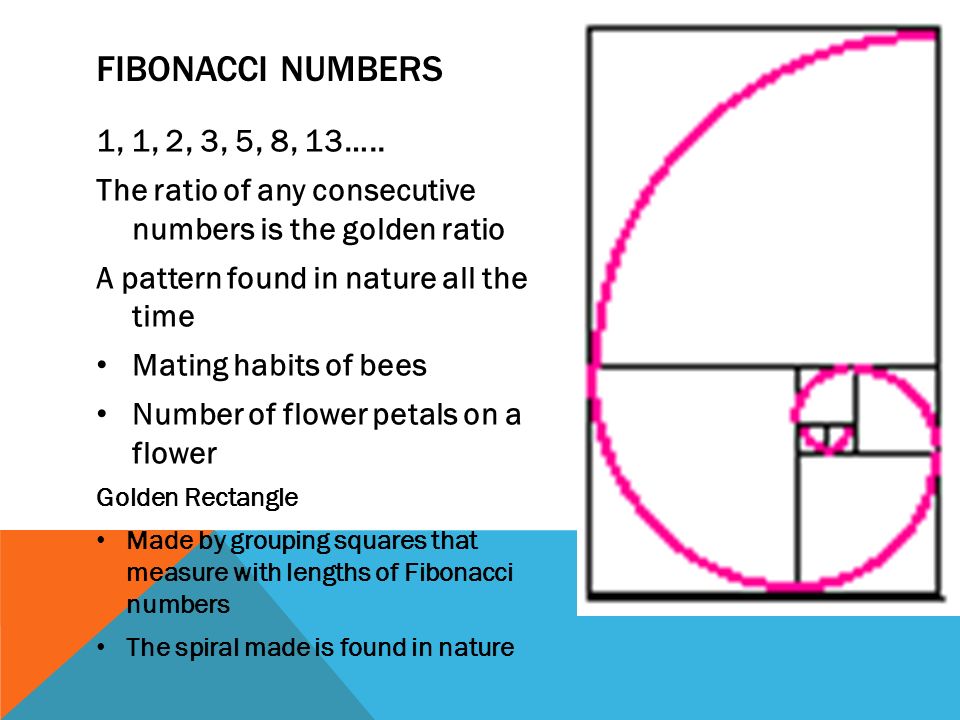
36+ 1 2 3 5 8 Sequence Background. , called the fibonacci sequence , such that each number is the sum of the two preceding ones, starting from 0 and 1. This free number sequence calculator can determine the terms (as well as the sum of all terms) of an arithmetic, geometric, or fibonacci sequence.
How do you use the geometric sequence of numbers 1, 2, 4, 8,…to find r, the ratio between 2 consecutive terms?
Moreover, because the common difference is d = 3 and the first term is a1 = 2, the formula must have the form. Start studying geometric sequences assignment. The first five terms are found by plugging in 1, 2, 3, 4, and 5 for n. For example, the fibonacci sequence, which starts {0, 1, 1, 2, 3, 5, 8.}, with each.